How long is a second?
There are a few ways to answer this question. The most reasonable response is ‘I don’t know, fuck off, why are you asking me this?’ The smartass response is that it’s a sixtieth of a minute. Neither of these is particularly satisfying.
[jwplayer qEJdAMqg]
A more satisfying response would be that it’s a sixtieth of a minute, which in turn is a sixtieth of an hour, which in turn is one twenty-fourth of the time that it takes for the Earth to complete a full rotation. This is more satisfying, but it’s also wrong, because the Earth’s rate of rotation is very slowly, er, slowing over time.
Because our understanding of just about everything has increased throughout history, we’ve had to slowly adapt our previously quite arbitrary units of measurement to definitions that are based on physical constants — known quantities that will never change (at least, not without invalidating our entire understanding of the universe).
The second, for example, has been formally defined since 1967 as “the duration of 9,192,631,770 periods of the radiation corresponding to the transition between the two hyperfine levels of the ground state of the caesium-133 atom“. Not terribly easy to remember or explain, but something that is not liable to change any time soon. Similarly, since 1983 the metre has been defined as precisely how far light travels in a vacuum in the space of 1/299,792,458 of a second.
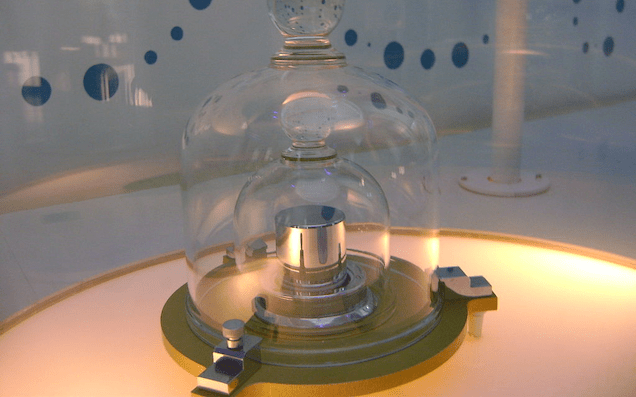
Until now, the kilogram has managed to avoid such modernisation. From the late 1800s right up until today, the kilogram has been defined as the exact weight of a lump of platinum and iridium called the International Prototype of the Kilogram, which sits locked in an underground vault in France. Now that lump of platinum and iridium has been rendered redundant by the kilogram’s integration into the new definition of the Planck constant.
We’re going to breeze through (and probably monstrously oversimply) some very tricky science stuff here, but bear with me. The Planck constant, like the speed of light, is a physical constant; we know its numerical value and that value doesn’t change. The definition of that value (6.62607015×10−34 kg⋅m2⋅s−1) contains the values for the metre and the second, both of which (as we mentioned previously) are also defined from physical constants and have fixed numerical values, which means we can then use all of these to derive the value of the kilogram.
The kilogram was the last of the International System of Units base units not to be defined as a product of a physical constant, meaning we no longer have to consult one canonical instance of any unit to check how long or how heavy or how fast it is.
Coming into effect today, the decision was made last year at the General Conference on Weights and Measures in Versailles, France, where it was passed with a unanimous vote.
The International Prototype of the Kilogram will, presumably, continue to sit there, slowly losing weight like it has been for the last hundred or so years.







